Graph

Theory

The code performs the follows the reasoning: for a chosen $a$ , $b$ and $c$ , select a random $x$ with $x \in [0, a)$ , $y$ with $y \in [0, b)$ and $z$ with $z \in [0, c)$ . If and only if the equation of the ellipsoid in standard form:

$\begin{eqnarray*} (\frac{x}{a})^{2} + (\frac{y}{b})^{2} + (\frac{y}{c})^{2} &\le& 1 \end{eqnarray*}$

is satisfied, then the generated $x$ and $y$ values describe a point $(x, y, z)$ which lies within the elipsoid's perimeter. Otherwise, select new values for $x$ , $y$ and $z$ and loop until the equation is satisfied.

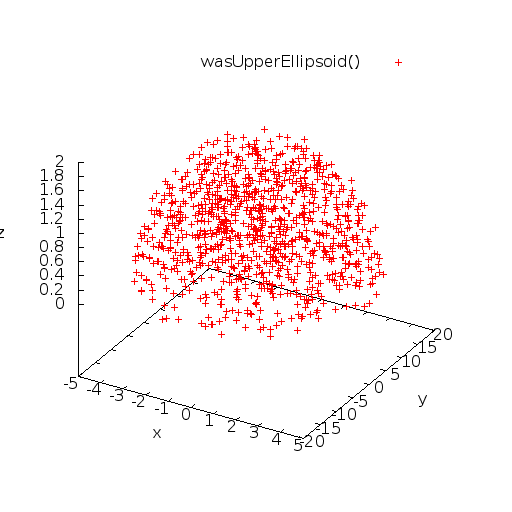
Since we want, in this case, an upper elipsoid, all $z$ values must be positive.

Code

wasUpperEllipsoid.lsl

///////////////////////////////////////////////////////////////////////////
//    Copyright (C) 2016 Wizardry and Steamworks - License: GNU GPLv3    //
///////////////////////////////////////////////////////////////////////////
vector wasUpperEllipsoid(float a, float b, float c) {
    float x = llPow(-1, 1 + (integer) llFrand(2)) * llFrand(a);
    float y = llPow(-1, 1 + (integer) llFrand(2)) * llFrand(b);
    float z = llFrand(c);
    if(llPow(x/a,2) + llPow(y/b,2) + llPow(z/c,2) <= 1)
        return <x, y, 0>;
    return wasUpperEllipsoid(a, b, c);
}

Table of Contents

Graph

Theory

Code