Chromatic Music Scale

An octave is a sequence of 12 notes, given by the recurrence relation:

$$ \begin{eqnarray*} f_{x+1} = f_{x} * \sqrt[12]{2^{x}} \end{eqnarray*} $$

with $x:\mathbb{N}\mapsto[0,11]$ and starting from the note $A$ with $f_{0}=440Hz$.

The following table is an octave table (sequence of pitches) with notes corresponding to frequencies. Each successive frequency is determined by multiplying the previously obtained frequency with $\sqrt[12]{2} \approx 1.05946$.

Note Frequency (Hz)
$A$ 440.00
$A\sharp$/$B\flat$ 466.16
B 493.88
C 523.25
$C\sharp$/$D\flat$ 554.37
D 587.33
$D\sharp$/$E\flat$ 622.25
E 659.26
F 698.46
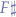$F\sharp$/$G\flat$ 739.99
G 783.99
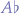$G\sharp$/$A\flat$ 830.61

the next frequency $880.00$ corresponds to the note $A$ in the next octave.